Density, distribution function, quantile function,
random generation and hazard function for the Beta Generalized Exponentiated distribution
with parameters mu, sigma, nu and tau.
Usage
dBGE(x, mu, sigma, nu, tau, log = FALSE)
pBGE(q, mu, sigma, nu, tau, lower.tail = TRUE, log.p = FALSE)
qBGE(p, mu, sigma, nu, tau, lower.tail = TRUE, log.p = FALSE)
rBGE(n, mu, sigma, nu, tau)
hBGE(x, mu, sigma, nu, tau)Value
dBGE gives the density, pBGE gives the distribution
function, qBGE gives the quantile function, rBGE
generates random deviates and hBGE gives the hazard function.
Details
The Beta Generalized Exponentiated Distribution with parameters mu,
sigma, nu and tau has density given by
\(f(x)= \frac{\nu \tau}{B(\mu, \sigma)} \exp(-\nu x)(1- \exp(-\nu x))^{\tau \mu - 1} (1 - (1- \exp(-\nu x))^\tau)^{\sigma -1},\)
for \(x > 0\), \(\mu > 0\), \(\sigma > 0\), \(\nu > 0\) and \(\tau > 0\).
Author
Johan David Marin Benjumea, johand.marin@udea.edu.co
Examples
old_par <- par(mfrow = c(1, 1)) # save previous graphical parameters
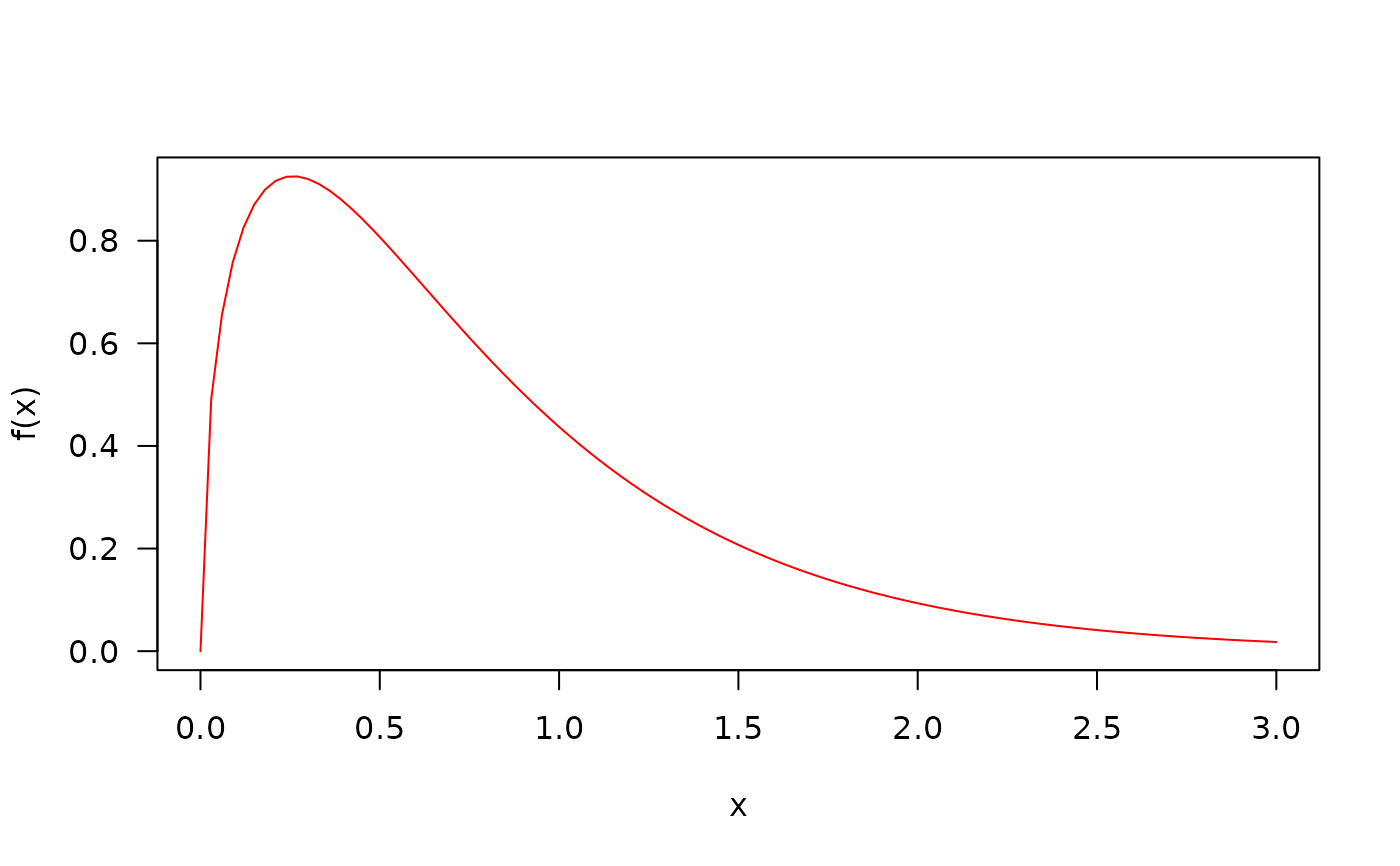
## The probability density function
curve(dBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1), from = 0, to = 3,
col = "red", las = 1, ylab = "f(x)")
 ## The cumulative distribution and the Reliability function
par(mfrow = c(1, 2))
curve(pBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1), from = 0, to = 6,
ylim = c(0, 1), col = "red", las = 1, ylab = "F(x)")
curve(pBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1, lower.tail = FALSE),
from = 0, to = 6, ylim = c(0, 1), col = "red", las = 1, ylab = "R(x)")
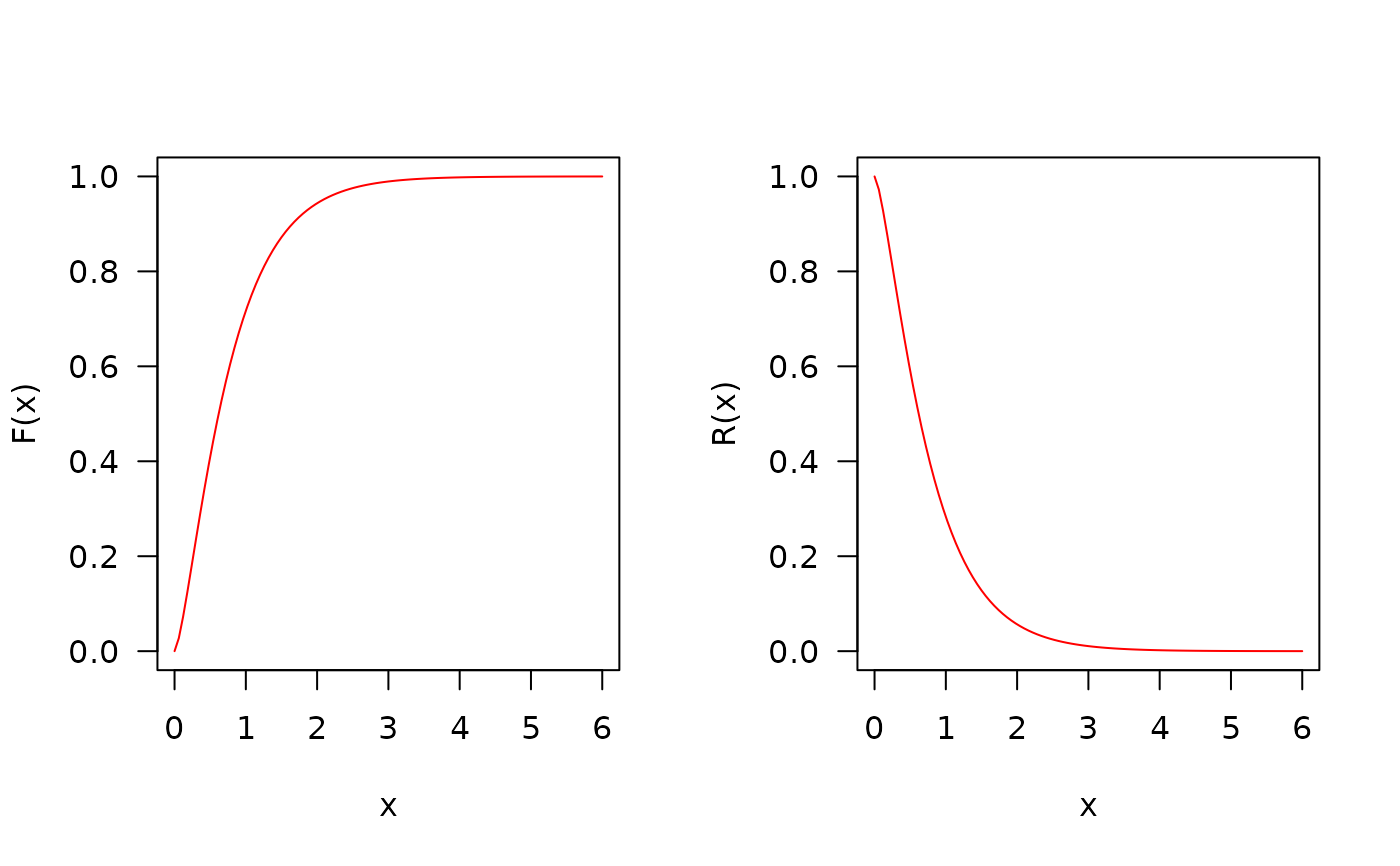
## The cumulative distribution and the Reliability function
par(mfrow = c(1, 2))
curve(pBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1), from = 0, to = 6,
ylim = c(0, 1), col = "red", las = 1, ylab = "F(x)")
curve(pBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1, lower.tail = FALSE),
from = 0, to = 6, ylim = c(0, 1), col = "red", las = 1, ylab = "R(x)")
 ## The quantile function
p <- seq(from = 0, to = 0.99999, length.out = 100)
plot(x = qBGE(p = p, mu = 1.5, sigma =1.7, nu=1, tau=1), y = p,
xlab = "Quantile", las = 1, ylab = "Probability")
curve(pBGE(x, mu = (1/4), sigma =1, nu=1, tau=2), from = 0, add = TRUE,
col = "red")
## The random function
hist(rBGE(1000, mu = 1.5, sigma =1.7, nu=1, tau=1), freq = FALSE, xlab = "x",
ylim = c(0, 1), las = 1, main = "")
curve(dBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1), from = 0, add = TRUE,
col = "red", ylim = c(0, 0.5))
## The quantile function
p <- seq(from = 0, to = 0.99999, length.out = 100)
plot(x = qBGE(p = p, mu = 1.5, sigma =1.7, nu=1, tau=1), y = p,
xlab = "Quantile", las = 1, ylab = "Probability")
curve(pBGE(x, mu = (1/4), sigma =1, nu=1, tau=2), from = 0, add = TRUE,
col = "red")
## The random function
hist(rBGE(1000, mu = 1.5, sigma =1.7, nu=1, tau=1), freq = FALSE, xlab = "x",
ylim = c(0, 1), las = 1, main = "")
curve(dBGE(x, mu = 1.5, sigma =1.7, nu=1, tau=1), from = 0, add = TRUE,
col = "red", ylim = c(0, 0.5))
 ## The Hazard function(
par(mfrow=c(1,1))
curve(hBGE(x, mu = 0.9, sigma =0.5, nu=1, tau=1), from = 0, to = 2,
col = "red", ylab = "Hazard function", las = 1)
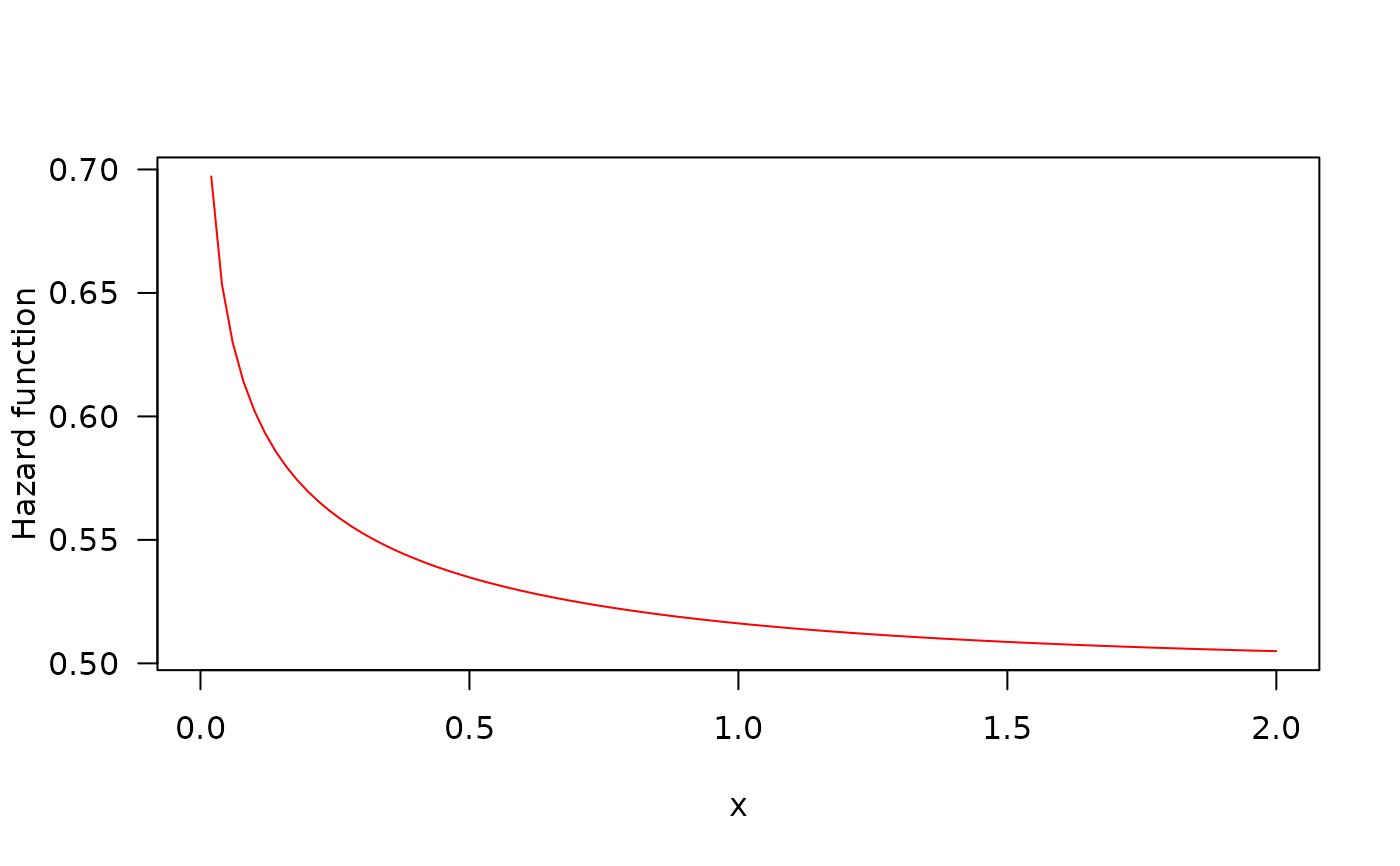
## The Hazard function(
par(mfrow=c(1,1))
curve(hBGE(x, mu = 0.9, sigma =0.5, nu=1, tau=1), from = 0, to = 2,
col = "red", ylab = "Hazard function", las = 1)
 par(old_par) # restore previous graphical parameters
par(old_par) # restore previous graphical parameters